and David Tingay6
(5)
Department of Pediatrics, Service of Neonatology and Pediatric Intensive Care, University Hospital of Geneva, CH-1211 Geneva 14, Switzerland
(6)
Department of Neonatology, Murdoch Childrens Research Institute, Royal Children’s Hospital, Flemington Rd., Parkville, VIC, 3052, Australia
Educational Aims
To understand what information can be gathered from respiratory system compliance measurements
To review various factors and error sources that might influence dynamic compliance measurements during ongoing mechanical ventilation
To understand why analysis of the shape of the dynamic PV curve can give more helpful information than analysis of respiratory system compliance values only
To understand the difficulties and limited value of respiratory compliance measurements during high-frequency ventilation (HFV)
During mechanical ventilation, respiratory mechanics can be assessed by studying the pressure–volume (PV) relationship, describing the elastic properties of the respiratory system, lungs and chest wall, above end-expiratory lung volume (EELV) or functional residual capacity (FRC). However, it is apparent that a complete description of the elastic behaviour of the respiratory system would require measurements made over the complete volume range, from residual volume (RV) to total lung capacity (TLC).
Analysis of the PV relationship can be used in two ways, first to describe the overall compliance of the respiratory system under quasi-static conditions, which might be used as an indicator of evolution of lung disease over time, and second for setting ventilator pressures within the range that is thought to be safe (i.e. above the lower (LIP) and below the upper inflection point (UIP) (for further information see Sect. 11.8). The segment between the LIP and the UIP represents a zone of best compliance within tidal ventilation should occur (Fig. 13.1). However, it has been realised that the real PV curve of the respiratory system, which describes respiratory system behaviour, has to be obtained during static or at least semi-static conditions (i.e. no flow conditions or low flow conditions, respectively) to avoid artefacts mainly due to various forces opposing inspiratory flow such as:
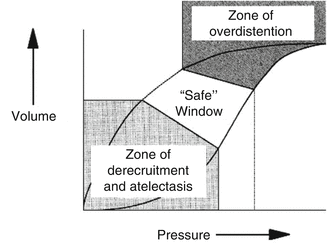
Fig. 13.1
Pressure–volume curve of a moderately diseased lung, such as one with adult respiratory distress syndrome. Two hazard zones exist. In the zone of overdistention, damage occurs from oedema fluid accumulation, surfactant degradation, high oxygen exposure and mechanical disruption. In the zone of derecruitment and atelectasis, lung injury develops through the direct trauma of repeated closure and reexpansion of airways and alveoli, by stimulation of the lung’s inflammatory response, by inhibition of surfactant, through the effects of local hypoxemia, and through compensatory overexpansion of the rest of the lungs as the lung ‘shrinks’. High end-expiratory pressures and small tidal volumes are needed to stay in the ‘safe’ window (Reprinted with permission from Froese (1997))
Viscous forces (friction along the endotracheal tube, the airways and lung tissue affecting flow)
Elastic forces (elastic forces by the lung and elastic recoil forces by the chest wall)
Viscoelastic forces (stress adaptation within lung and chest wall tissues)
Inertial forces (at the start of inspiration and expiration)
Additional factors that will induce additional errors in PV measurements are:
Compressibility of gases
Gas resorption in the lungs
Inertial forces (at the beginning of both, inspiration and expiration)
Inhomogeneity within the lungs with inhomogeneous filling behaviour of various lung regions
Generation of the static or semi-static PV curve on bedside is cumbersome using either step-by-step or occlusion inflation/deflation methods, alternatively continuous slow flow inflation methods have been proposed (Sydow et al. 1991, 1993; D’Angelo et al. 1994; Ranieri et al. 1994; Lu et al. 1999). All methods require the patient being sedated and muscle paralysed. The advantage of this static and semi-static measurements is, because of pressure and volume readings, made under no flow conditions and in steady state condition of the system (i.e. only when a stable pressure reading is reached) for the step-by-step inflation and deflation method by a super-syringe using small volume aliquots or during no flow for the flow interruption techniques during constant low flow inflation (Lu et al. 1999) that airway resistance does not, or almost not, influence measurements of the respiratory systems PV curve that describes the behaviour of the respiratory system over the whole volume range, i.e. form functional residual capacity to total lung capacity.
In cooperative subjects, it is possible to measure the PV curve starting at RV, which influences the shape of the inflation, but not of the deflation, PV curves. Likewise, in an excised lung, PV curves can be obtained from the degassed state, and this has a profound effect on the shape of the inflation limb of the PV curve, while the deflation limb remains unchanged. In other words, the shape of the inflation limb depends on the initial lung volume at which its determination starts (Jonson et al. 1999). However, making measurements from RV is impractical and indeed dangerous in patients with acute lung injury. Therefore, measurements of PV curves are generally made starting from end-expiratory lung volume (EELV) at 0 cm H2O airway pressure. In normal subjects, EELV is synonymous with FRC, defined as the volume determined by the balance of static passive forces of the lung and chest wall (see Fig. 11.23, Sect. 11.8).
By definition, positive pressure ventilation with a PEEP value above 0 cm H2O in a sedated and paralysed patient occurs above FRC. Therefore, with any setting on the ventilator, only the volume above the FRC can be modified according to the characteristics of the PV curve (i.e. compliance and hysteresis). In some patients the FRC is virtually at the RV; in others there is a substantial expiratory reserve volume. If the disease is associated with a highly compliant lung or chest wall, the FRC will be high, and, conversely, if the lung or chest wall compliance is low, the FRC will be low. In the former case, one may not see an inflection point on the inflation limb of the PV curve, whereas it will probably be present in the latter. The position of the FRC within the TLC is thus ‘disease specific’ and the problem is that even when FRC is measured with a gas dilution technique, it is difficult to assess it in relation to the TLC. Furthermore, in ALI/ARDS there is a significant portion of the intrathoracic gas volume occupied by tissue oedema, fluids and inflammatory cells, as evidenced by computed tomographic studies; therefore lung gas volume measurements may not always be predictive of total thoracic volume (Gattinoni et al. 1998). A further difficulty is that if by some ventilatory strategy, although one succeeds in increasing lung volume, one is never sure whether this is due to recruiting new gas exchange units (good) or overdistending already open units (bad) (Rouby et al. 2002).
Furthermore, for the purpose of such measurements, it would be necessary to interrupt the normal ventilatory pattern of the patient, which does require the patient to be passive, which often means to be paralysed (for further information see Sect. 11.8).
13.1.1 Dynamic Pressure–Volume-Loop Analysis and Dynamic Compliance for Assessment of Disease Evolution
Static or semi-static methods of PV measurements may offer a diagnostic tool (Bone 1976) diagnosis of causes for acute respiratory distress syndrome (i.e. extrapulmonary versus intrapulmonary ARDS) allowing to describe progress of disease (Falke et al. 1972; Suter et al. 1975; Matamis et al. 1984) or document the effect of treatment or an intervention (Lachmann et al. 1982; Tooley et al. 1987) (Pfenninger et al. 1992); the latter can be of special interest for various clinical research purposes and eventually allow for prognosis (Gattinoni et al. 1984), but they cannot be used for continuous monitoring of respiratory mechanics during ongoing ventilation.
13.1.2 Dynamic Pressure–Volume-Loop Analysis and Dynamic Compliance Measurements for Choosing Ventilator Settings
13.1.2.1 During Conventional Ventilation
There exists a certain interest in using measurements and analysis of respiratory mechanics during ongoing mechanical ventilation that could help to improve ventilator settings. However, mechanical properties of the respiratory system assessed under the dynamic condition of mechanical ventilation are not equivalent to those assessed under static conditions (Stahl et al. 2006). The observations that (1) the inflation limb of the quasi-static PV curve (as generated by the super-syringe or low flow inflation methods) is distinctly different from the deflation limb, (2) the inflation limb is highly sensitive to the initial inflation conditions (i.e. whether inflation starts from zero PEEP or another PEEP level) (Jonson et al. 1999) and preceding lung history (i.e. whether the patient was disconnected or even suctioned shortly before), and (3) the tidal cycle is not placed on the inflation limb of the quasi-static PV (Rimensberger et al. 1999) illustrate well how difficult it can be to use the slope of the tidal cycle PV curve (i.e. dynamic compliance) during titration of PEEP stepwise upwards as only indicator for best ventilator settings as suggested many years ago (Suter et al. 1975, 1978). The following strategies might potentially help, despite several limitations of each method, to optimise PEEP and V t or plateau or peak pressure setting, respectively.
13.1.2.1.1 Optimising PEEP by Dynamic Compliance Measurements and Graphic Analysis
Classically, as cited in several textbooks, it has been thought that changes in dynamic compliance values during upward titration of PEEP would clearly indicate the best PEEP level given the concept that the tidal cycle would move along the static inflation PV curve of the respiratory system (Fig. 13.2). With the recognition that PV hysteresis of the respiratory system exists (Escolar et al. 2002; Escolar and Escolar 2004) (see also Sect. 11.8), that the deflation limb of the static PV is much more stable and therefore relatively independent from lung history (i.e. previous inflation conditions) (Rimensberger and Bryan 1999) and that after a lung recruitment the dynamic PV cycle during ongoing ventilation can be placed on the deflation limb of the corresponding static PV curve (Fig. 13.3), it has been suggested to use a decremental PEEP following a recruitment manoeuvre to indentify optimal PEEP settings (Rimensberger and Bryan 1999; Hickling 2001; Suarez-Sipmann et al. 2007; Maisch et al. 2008). The PEEP that resulted in the highest compliance during decremental PEEP steps is considered to be optimal (Fig. 13.4). Furthermore, it has been shown that changes in aerated and non-aerated lung volumes induced by a lung recruitment manoeuvre can be adequately represented by respiratory compliance but much less by changes in oxygenation or shunt (Henzler et al. 2005). This approach of using the individual patient’s lung mechanics to open the lung and set PEEP is very promising because of its ease for implementation (Hermle et al. 2002).
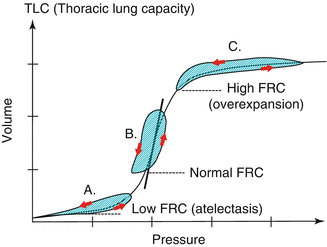Fig. 13.2
Classically, as cited in several textbooks and company handbooks, it has been thought that changes in dynamic compliance (indicated by the slope of the ventilatory PV-cycle in green color) would clearly indicate the best PEEP level (in this example scenario B) given the concept that the tidal cycle would move along the static inflation PV-curve of the respiratory system. This does not take in account hysteresis of the respiratory system and is therefore over simplified. The ventilatory tidal cycle never lies on the inflation limb of the static PV-curve (see also Fig. 13.3)
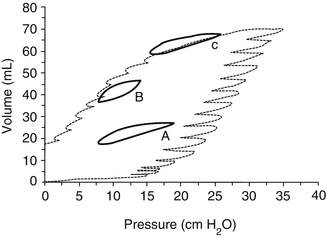Fig. 13.3
Dynamic loops (tidal volume, 5 mL/kg) with 3 PEEP settings with and w/o lung recruitment inscribed into the quasi-static pressure/volume curve of the respiratory system of one animal after surfactant depletion with repeated lung washes: (A) PEEP < P inf without preceding SI; (B) PEEP < P inf after a SI; (C) PEEP > P inf. Note that the dynamic loop A (PEEP without any sustained inflation) is positioned above the inflation limb of the static PV curve and that loop B (PEEP after sustained inflation) is positioned on the deflation limb of the static PV curve. With PEEP > P inf, the dynamic loop (C) is on the asymptote of the static PV curve. PEEP positive end-expiratory pressure, P inf lower inflection point on the inflation limb of the overall PV curve, SI sustained inflation (Reprinted with permission from Rimensberger et al. (1999))
Fig. 13.4
Individual changes in dynamic compliance decremental PEEP steps after a recruitment manoeuvre (RM) in an experimental study. A biphasic response (i.e. a first increment and a subsequent decrement with a visually identifiable maximum) can be observed. Each symbol represents an individual animal. RM recruitment manoeuvre, PEEP positive end-expiratory pressure (Modified with permission from Suarez-Sipmann et al. (2007))
Alternatively, the lower and upper inflection points of the quasi-static PV curve were regarded repeatedly as points of interest to avoid cyclic derecruitment and overdistension and to optimise ventilatory settings (Fig. 13.5). However, this concept is not fully appropriate since it has been clearly shown that alveolar reinflation occurs along the whole P/V curve. In addition, the dynamic tidal cycle is positioned always above the inflation limb of the quasi-static PV curve (Rimensberger et al. 1999; Hickling 2001) (Figs. 13.6 and 13.7), and this is more marked when PV hysteresis is important. Therefore, the lower inflection point has no relationship with alveolar opening and closure and does not indicate the positive end-expiratory pressure needed to prevent alveolar collapse (Maggiore et al. 2003). In short, identification of a lower inflection point on the dynamic pressure–volume loops obtained during tidal ventilation should not be used to guide PEEP settings (Adams et al. 2001) (see also Sect. 11.8.2).
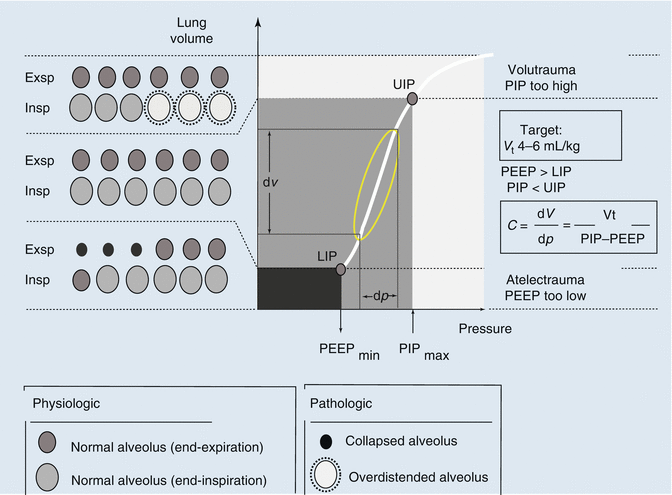Fig. 13.5
The classical concept of the interpretation of the pressure–volume curve indicates that with positioning the tidal cycle by using a PEEP value above the lower inflection point (LIP), the risk for atelectrauma should be reduced, and with positioning the tidal cycle by using a PIP value below the upper inflection point (UIP), the risk for volutrauma should be reduced. PEEP positive end-expiratory pressure, PIP positive inspiratory pressure, LIP lower inflection point, UIP upper inflection point, C compliance, dV delta volume = tidal volume, dp delta pressure, V t tidal volume (With permission, modified from Berger and Stocker (2004))
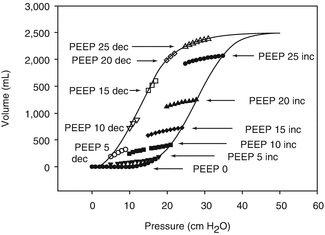Fig. 13.6
Inspiratory tidal PV plots with V T 140 ml with incremental (black symbols) and decremental (open symbols) PEEP levels from 0 to 25 cm H2O derived mathematically from multiple units lung model. At each PEEP level, the volume at equivalent pressures and the mean tidal PV slope (i.e. tidal cycle compliance or dynamic compliance during conventional mechanical ventilation) are greater during decremental PEEP. With zero PEEP, the plots for incremental and decremental PEEP are superimposed. Note that the dynamic tidal PV cycle is never positioned on the plotted inflation limb of the overall PV curve (Reprinted with permission from Hickling (2001))
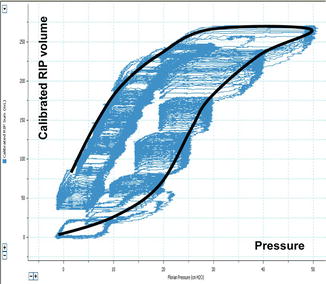Fig. 13.7
Tracing of the dynamic pressure–volume curve (blue line) during incremental and decremental PEEP steps using volume-calibrated respiratory inductive plethysmography (RIP) in an animal after surfactant depletion by repeated lung washes and superimposed the theoretical quasi-static PV curve (black line). Note that the lower inflection point on the static PV curve (i.e. PV envelop) has no relationship with alveolar opening and closure and does not indicate directly the positive end-expiratory pressure needed to open the lung or to prevent alveolar collapse
However, airway graphics can, despite the limitations mentioned above, be useful to guide ventilation.
1.
One possible strategy is to use airway graphics to determine ‘best’ PEEP (Fig. 13.8) in order to avoid airway collapse end-expiration, as testimonied by a flat horizontal dynamic pressure–volume (PV) curve (0 volume reading between the set PEEP and a higher pressure level at which airway opening will start to happen). Whereas when the first part of the PV curve from set PEEP shows a slow and a second part a fast increase in volume (Fig. 13.8), this will not indicate that PEEP settings are too low and therefore allowing for collapse. Such a phenomenon is a result of initial fast flows in a high resistive system (endotracheal tube) during rapid increase of airway pressures, because pressures are measured in the respiratory circuit at the ETT connection (i.e. before the part of high resistance in the respiratory circuit that includes the endotracheal tube) and not on the tracheal level (Karason et al. 2000) (see Fig. 11.27). Therefore, the dynamic PV loop cannot give a clear indication on the required PEEP level. However, a completely flat and horizontal line for the first part of the inflation limb might suggest that there might be no volume change induced when pressures are increased which could indicate that there is major lung collapse during the first part of lung inflation. Whereas when the first part of the PV curve from set PEEP shows a slow and a second part a fast increase in volume. The change in curvature of the inflation limb is related mainly to endotracheal tube resistance. This latter does not indicate that PEEP settings are too low. This can be easily confirmed by the observation that this ‘lower inflection point’ is shifted to the right when PEEP is increased (Fig. 13.8, see also Fig. 11.27 in Sect. 11.8).
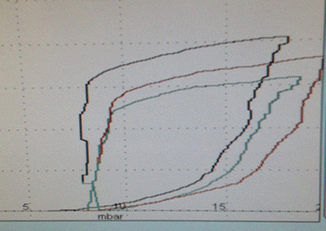Fig. 13.8
Dynamic pressure loop as displayed by the SLE 5000 ventilator in the TCPL mode. PEEP and PIP are increased from 6 to 8 and PIP from 18 to 20 cm H2O within three breath cycles. Note the shift of the lower inflection point (LIP) from 14 cm H2O with a PEEP setting of 6 cm H2O (black line) to 16.5 cm H2O with a PEEP setting of 8 cm H2O (red line). The green line depicts the respiratory cycle occuring during changes of settings are made. The appearance of a LIP is a result of airway resistance during fast flow inflation and does not indicate lung opening. TCPL Time cycled pressure limited
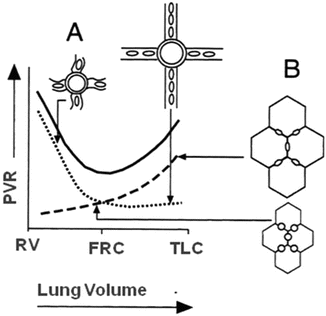Fig. 13.9
Pulmonary vascular resistance (PVR) is lowest at functional residual capacity (FRC). In the diseased lung, FRC is in general reduced due to lung infiltration, consolidation, atelectasis or presence of interstitial oedema, with resultant loss of pulmonary vascular integrity and increased PVR (A). Any recruitment in lung volumes in order to restore as much as possible normal FRC will therefore lower PVR, whereas overdistention of lung units will again increase PVR through compression of the pulmonary vascular bed (B)
2.
On the other side of the PV range, pulmonary overdistension occurs when the volume limit of some areas of the lungs is approached. It will be manifested as a dramatic reduction in compliance at the terminal end of the breath. This commonly is referred to as ‘beaking’ and often numerically expressed as by the C20/C value (i.e. the ratio between the slope, i.e. compliance of the last 20 % of the upper pressure range and the slope, i.e. compliance of the total PV loop) (see Fig. 11.51). Overdistension should be avoided as it has several deleterious effects on the cardiovascular and pulmonary system. First, overdistension puts the patient at risk for baro-/volutrauma. Second, overdistension can increase dead space and therefore render ventilation less efficient. Third, overdistension will result in a dramatic increase in pulmonary vascular resistance (Fig. 13.9) from pulmonary vascular compression and a resultant reduction in cardiac output. These effect will be even more dramatic at higher levels of PEEP while maintaining identical V t or driving pressure (ΔP = PIP − PEEP). When terminal ‘beaking’ is observed, the clinician should reduce the set PIP or V t to reduce the risk of overdistension of some lung areas.
3.
A third concept would be the analysis of the curvature of the pressure–time curve generated during a low flow inflation for V t delivery. Ranieri et al. have shown that this might allow to detect variations in compliance during tidal inflation, indicating either the occurrence of alveolar overdistention at end-inspiration, or alveolar recruitment during tidal inflation (Figs. 13.10 and 13.11) (Ranieri et al. 2000). Hammer and colleagues measured FRC in relation to TLC by gas dilution in children with a variety of lung disease. Determination of TLC and FRC (i.e. the FRC/TLC ratio) allowed estimating the degree of lung overinflation (Hammer et al. 1998). However, for bedside application this latter method is not as simple to be used.
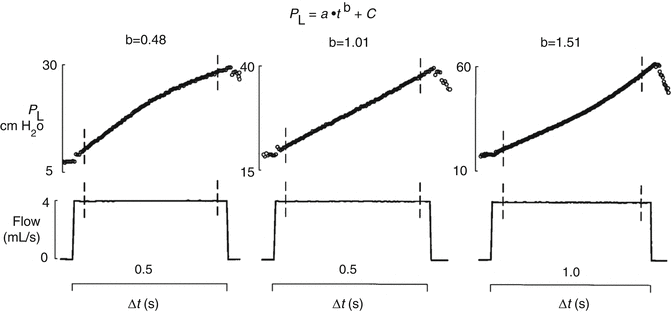Fig. 13.10
The conceptual illustration of the dynamic pressure–time (P–t) curve. Based on the power equation P L = a ⋅ t b + c, b = 0.5 produces a convex P–t curve, indicating continuing recruitment; b = 1 produces a straight P–t line, indicating no alveolar continuing recruitment or overdistension; and b = 1.5 produces a concave P–t curve, indicating alveolar overdistension. The power equation was applied to the transpulmonary pressure (PL) signal during a constant inspiratory flow (vertical bars) (Reprinted with permission from Ranieri et al. (2000))
Fig. 13.11
Representative data record showing physiologic variables (flow, transpulmonary pressure (PL) and changes in lung volume (ΔV)) and the appearance at end-expiration of the excised lung during the different experimental conditions. The equation P L = a ⋅ t b + c was applied to the inspiratory PL during constant flow (vertical bars on flow and PL). Low-volume stress, coefficient b = 0.5, indicates ongoing recruitment; minimal stress, b = 1, indicates no ongoing recruitment or overdistention; and high-volume stress, b = 1.5, indicates overdistension. PEEP positive end-expiratory pressure (Reprinted with permission from Ranieri et al. (2000))
13.1.2.1.2 Identification of Dynamic Hyperexpansion
When airway resistance is high and/or fast respiratory rates are used, the patient is at risk for dynamic hyperexpansion. This occurs when exhalation does not come to completion. As a result there is retained gas in the patient’s respiratory system (i.e. ‘gas trapping’) leading to intrinsic PEEP, which is difficult to control and should be if any possible avoided. Dynamic hyperexpansion might decrease venous return and hence preload and cardiac output. To limit the risk for dynamic hyperexpansion, one should decrease the T I, which if becoming inappropriately short may decrease delivered V t (see above) or reduce the ventilatory rate to allow for a longer T E.
13.1.2.2 During High-Frequency Ventilation
13.1.2.2.1 Monitoring of Compliance During High-Frequency Ventilation
There is substantial animal evidence to suggest that static compliance (Crs) can be used to determine the optimal application of high-frequency ventilation (HFV). Byford et al. (1988) showed that improvements in static and ‘quasi-static’ CRS were directly related to the magnitude of mean airway pressure (P aw) change, and subsequent recruitment achieved, after sustained inflations during HFV in surfactant-deficient rabbits. Later, Bond and Froese (1993) also reported, in the same animal model, an improvement in Crs after application of a volume recruitment manoeuvre during HFV. The deflation limb of the pressure–volume relationship has been repeatedly shown to coincide with the point of optimal static Crs during HFV (Rimensberger et al. 1999, 2000a; Goddon et al. 2001; McCulloch et al. 1988; Pillow et al. 2004). Finally, Wood et al. (2002) were able to identify atelectasis and overdistension on the inflation limb using static Crs. Monitoring of static Crs in patients receiving HFV is difficult as it requires cessation removal from tidal ventilation. Monitoring of dynamic Crs is problematic during HFV. The reasons for this are outlined below.
13.1.2.2.2 Difficulties in Determining Dynamic Crs During High-Frequency Ventilation
Despite the potential benefits of understanding the mechanical properties of the respiratory system during high-frequency ventilation, direct monitoring of dynamic Crs and resistance (R RS) is rarely performed at the bedside. This is due to the difficulties in determining both parameters during HFV. HFV is characterised by high flow, low tidal volume, low intra-alveolar change in pressure and high rate conditions, a situation that limits measurement conditions due to lower signal to noise ratio. It is worth considering the other reasons that limit monitoring of dynamic Crs during HFV.
13.1.2.2.3 Wave Form Attenuation
Unlike conventional modes of ventilation, change in pressure at the airway opening (ΔP) cannot be considered equal to change in transpulmonary pressure (P TP) during HFV (Gerstmann et al. 1990). Attenuation of waveform characteristics is not constant and influenced by multiple factors, including the resistance in the endotracheal tube and lung mechanics of the airways, lung tissue and chest wall (Pillow et al. 2002). Increasing alveolar compliance reduced the attenuation while increasing chest wall resistance increased it. Most modern HFV are limited to the measurement of airway pressure and flow at the airway opening; during HFV these measurements can only be considered proxies of the intrapulmonary mechanics.
13.1.2.2.4 Mathematical Assumptions of Respiratory System Mechanics
All commonly applied bedside methods of determining Crs, and RRS (see also Sects. 11.8 and 13.2) during mechanical ventilation apply mathematical assumptions to model the complex mechanical behaviour of the respiratory system. These assumptions are generally not valid during HFV or require computations beyond the capacity of most modern ventilators.
Although the Mead–Whittenberger analysis (Mead and Whittenberger 1953) is an accepted method of calculating Crs during mechanical ventilation, it cannot be applied to HFV, given the fact that there are no points of a zero flow condition attained at any point of the oscillatory cycle (Fig. 13.12). Similarly, the Least–Squares Regression Method applies the general equation of motion to a single compartment model (Stocks et al. 1996). Again, analysis has to be between two points of the pressure–volume curve, in this case taken from slope of the line joining the maximal and minimal volume. Again, the complex nature of pressure and flow waves, and attenuation, limits this methodology during HFV.
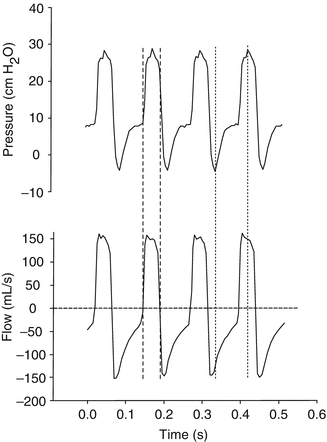
Fig. 13.12
Airway pressure (cm H2O) and flow (mL/s) waveforms measured at the airway opening during four oscillatory cycles (Sensormedics 3100A high-frequency oscillatory ventilator) in a term infant with meconium aspiration syndrome. The Mead-Whittenberger method of determining dynamic C rs cannot be applied as the two points of zero flow during each oscillatory cycle (dashed lines) do not correlate with the peak and trough of the pressure wave form (dashed lines)
In contrast, the multiple linear regression technique uses the entire respiratory cycle to calculate Crs (Stocks et al. 1996). This method is valid during the complex pressure and flow conditions of HFV but requires an analytic process that involves applying an algorithm to minimise errors between the observed pressure changes and the assumed pressure behaviour using the equation of motion for the flow and volume measurements. Multiple linear regression is currently impractical outside of a research environment.
13.1.2.2.5 Alternatives to Crs During HFV to Describe Respiratory Mechanics
In a state where the resistance of the ETT and ventilator circuit, lung disease, flow and applied ΔP are constant, it stands to reason that any change in measured HFV V T (V THFV) at the airway opening would be due to a change in Crs (Rossing et al. 1981; Slutsky et al. 1981; Wright et al. 1981). V THFV can be measured at the airway opening using pneumotachography (Boynton et al. 1989; Dimitriou et al. 1998; Kamitsuka et al. 1990; Scalfaro et al. 2001; Watson and Jackson 1984; Zimova-Herknerova and Plavka 2006) and correlated well to plethysmographic measurement of tidal volume (Courtney et al. 1990). This method has been shown to be feasible and accurate in adults receiving HFOV for ARDS (Hager et al. 2007; Hager et al. 2006). A relationship between P aw, volume state of the lung, lung volume and stroke volume (i.e. tidal volume during high frequency ventilation; V THFV) V THFV has been identified in 15 preterm infants with acute RDS receiving HFOV during the application of an open lung ventilation strategy to map the pressure–volume relationship of the lung (Miedema et al. 2012). Electrical impedance tomography measures regional end-expiratory thoracic volume and tidal volumes during HFOV, quantifying that V THFV changes at the airway opening were indicative of regional lung recruitment, overdistension and collapse. This suggests that V THFV is at least functionally representative of CRS. Measurement of tidal volume at the airway opening may be a useful relative indicator of the alveolar tidal volume during HFOV.
Stay updated, free articles. Join our Telegram channel
Full access? Get Clinical Tree
